
If we switch from one way of doing something to another merely because we are unsuccessful, it’s very likely that we do better the next time even if the new way of doing something is equal or worse. Chance is likely to contribute less on the second time we measure performance.
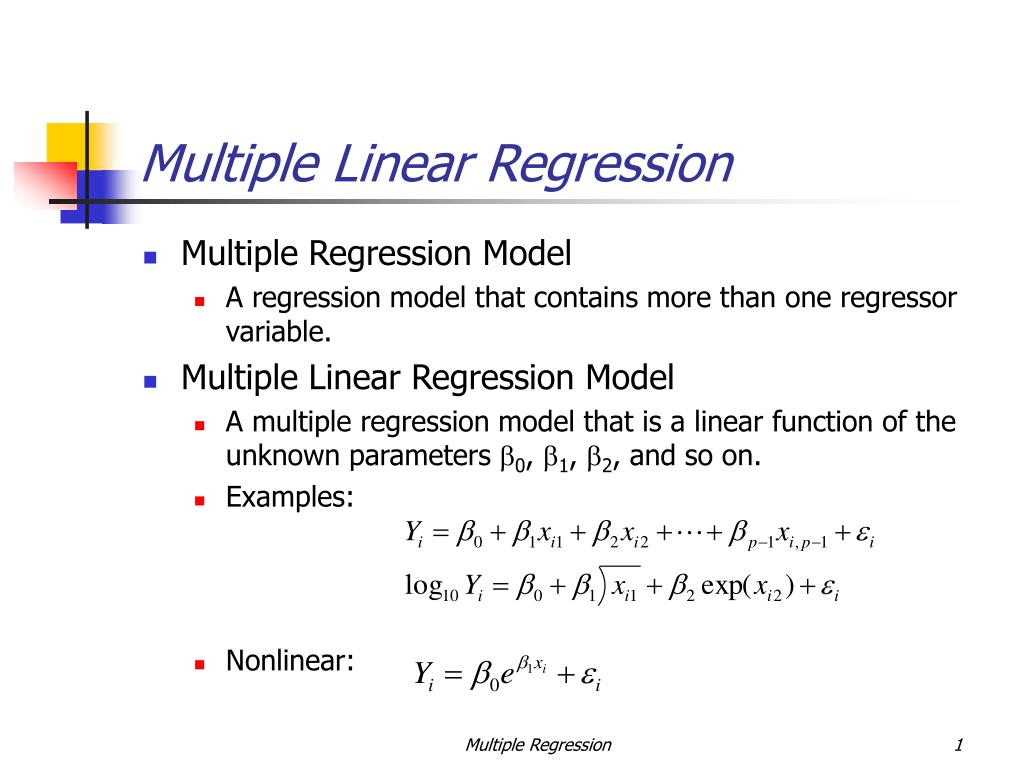
When the measurements are extreme, they are likely to be partly caused by chance. All measurements are made up of one true part and one random error part. Why? Testing measurements can never be exact. Extreme performance tends to get less extreme the next time. Our performance always varies around some average true performance. Their true ability perhaps hasn’t changed. And there could be many reasons for their earlier performance - stress, fatigue, sickness, distraction, etc.

Since these individuals were measured as being on the low end of the scale of skill, they would have shown an improvement even if they hadn’t taken the skill-enhancing program. Their higher scores could be the result of regression to the mean. John’s conclusion: “The skill-enhancing program caused the improvement in skill.” This isn’t necessarily true. Their scores are now higher than they were on the first test. In Seeking Wisdom, Peter Bevelin offers the example of John, who was dissatisfied with the performance of new employees so he put them into a skill-enhancing program where he measured the employees’ skill: The rule goes that, in any series with complex phenomena that are dependent on many variables, where chance is involved, extreme outcomes tend to be followed by more moderate ones. The notion of regression to the mean was first worked out by Sir Francis Galton. One of the errors that he examines in Thinking Fast and Slow is the infamous regression toward the mean. It turns out there is a whole set of logical errors that we commit because our intuition and brains do not deal well with simple statistics. Nobel prize-winning psychologist Daniel Kahneman wrote a book about biases that cloud our reasoning and distort our perception of reality. Learning about regression to the mean can help us.
It is important to minimize instances of bad judgment and address the weak spots in our reasoning. Learning to recognize when regression to the mean is at play can help us avoid misinterpreting data and seeing patterns that don’t exist. Regression to the mean is a common statistical phenomenon that can mislead us when we observe the world.


 0 kommentar(er)
0 kommentar(er)
